The Three Famous Math Problems Solved With Simple Concepts

Along with the flow of time, mankind has been fascinated with Mathematics for countless times. Sometimes, there are coincidences between numbers and their applications that are incredibly neat, while there are some extremely deceptively simple ones that still stump today’s computers. And the following three math problems are among those that were solved, fortunately, using two simple concepts.

1. Fermat’s Last Theorem

Pierre de Fermat, in 1637, scribbled a note in his Arithmetica copy. In particular, he wrote that if n>2 (n is an integer), there won’t be any whole number solutions to the equation: aⁿ+ bⁿ = cⁿ. He wrote a proof for the special case where n=4, with a claim that there’s a simple, “marvelous” proof to make this statement true for every integer. However, Fermat kept his mathematic endeavors in secret, and it was not until his death in 1665 that his conjecture was discovered.

No one could find a trace of the proof he claimed to have for all integers, so people raced to prove his conjecture. In the next over three centuries, a lot of great mathematicians like Legendre, Hilbert, and Euler stood and fell at Fermat’s Last Theorem. Some of them could find proof for more special cases, for example, n=14, 10, 5, and 3. But proving just special case misled the mathematicians from the real question: proving for all numbers.

They started doubting that some existing techniques could help prove the conjecture. Then in 1984, mathematician Gerhard Frey wrote the similarity that this theorem had with a geometrical identity called elliptical curve. With this in mind, mathematician Andre Wiles secretly worked on the proof in 1986. In 1995, with the help of his former student, Wiles managed to publish a paper proving the theorem through the use of the Taniyama-Shimura conjecture. After 358 years, Fermat’s Last Theorem was finally laid to rest.

2. The Enigma Machine

At the end of WWII, German engineer Arthur Scherbius developed the Enigma machine, which was most famous for encoding messages in the German military before and during the war.

This machine used rotors to rotate whenever a keyboard key was pressed so that each time a letter was sued, the Enigma substituted it with a different letter. For instance, the first time a B was pressed, a P was substituted, the second time a G, etc. It’s important to note that a letter would never appear as itself and there was no unsubstituted letter. Using the rotors created extremely precise, mathematically driven message ciphers which were nearly impossible to decode.

Initially, the machine had three substitution rotors, and in 1942, the fourth rotor was added for military use. The Allied Forces intercepted a few messages but they couldn’t decode them.

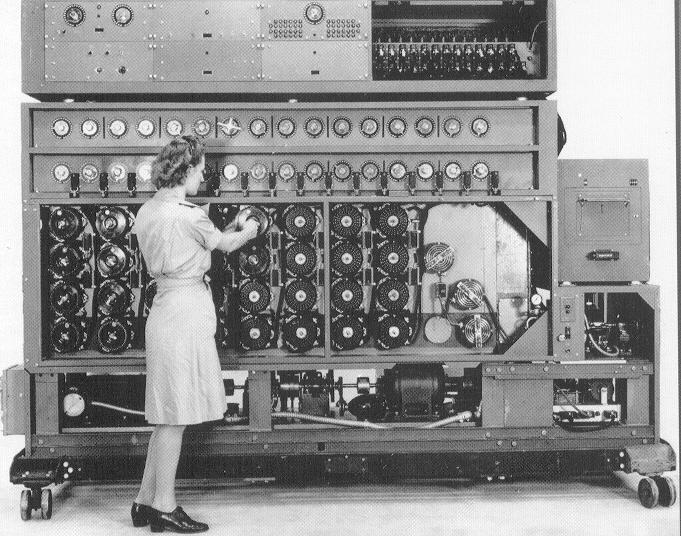
Alan Turing, a mathematician considered the father of computer science, figured out that the messages sent by the Enigma had a specific format: first, the message listed settings for the rotors. Once the settings were done, the message could be encoded on the receiving end. Turing built Bombe, a machine that tried many various combinations of rotor settings, it was able to statistically eliminate lots of legwork in message decoding. While the Enigma is as large as a typewriter, the Bombe is about 1.5 meters high, 1.8 meters long, and 0.3 meters deep. Estimations said that the Bombe cut the wartime down by two years.

3. The Four-Color Theorem

This theorem first surfaced in 1852. Francis Guthrie was coloring a map of England’s counties when he noticed that he needed only four colors at most to avoid having to counties next to each other in the same color. The conjecture was initially credited in publication to a University College professor who taught Guthrie’s brother. Thought the theorem worked, proving it was difficult. In 1879, mathematician Alfred Kempe wrote a proof for this conjecture, regarded as correct until another mathematician disproved it in 1890.

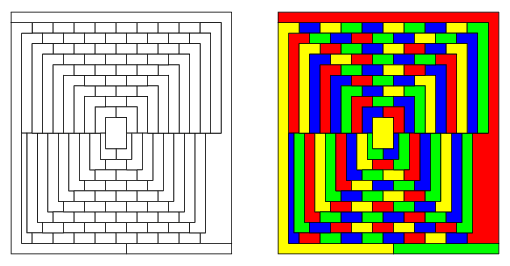
By the 1960s, Heinrich Heesch, a German mathematician, was using computers to solve different math problems. Two mathematicians from the University of Illinois, Wolfgang Haken and Kenneth Appel, decided to use Heesch’s methods for the problem. With that, the four-color theorem was the first to be proved using computers in 1976 by Haken and Appel.