This Mathematician Found A Much Simpler Way To Solve Quadratic Equations
Aadhya Khatri - Dec 27, 2019
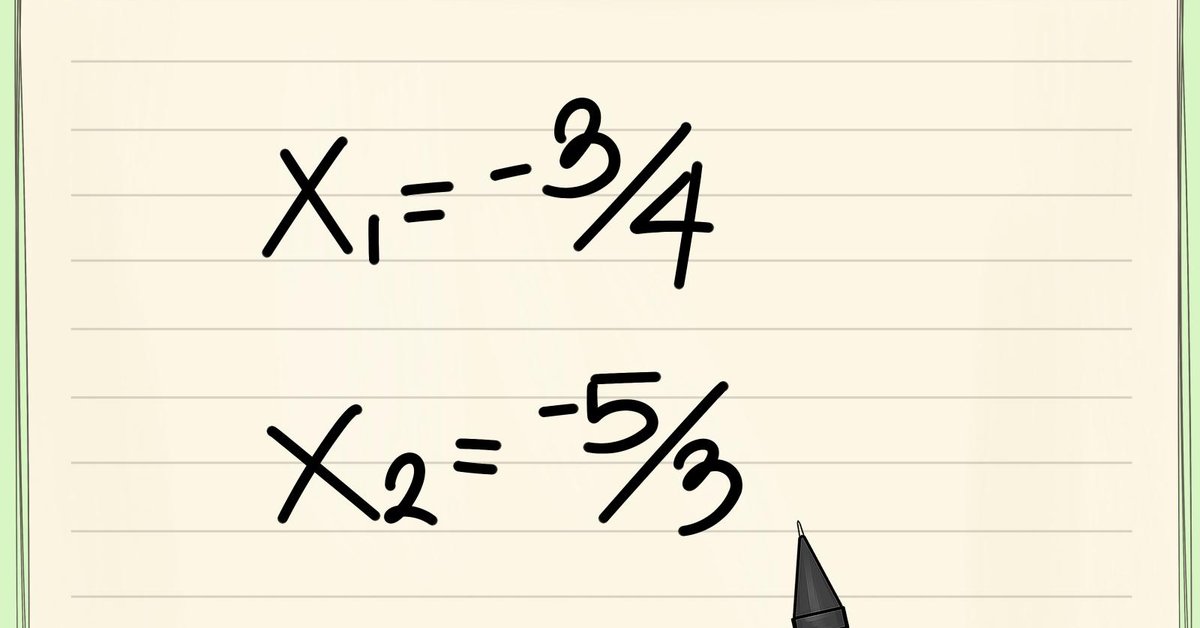
When you determine the value of z according to the alternative method of Loh, you can solve almost all quadratic equations
- Shakuntala Devi Awarded Guinness World Records’ Fastest Human Computation Certificate After 40 Years
- This Magical Number Will Help You Find A Parking Space, The Perfect Apartment, And Even A Suitable Spouse
- Can You Answer This Simple Math Problem That Has Tricked Einstein?
The quadratic formula is quite familiar to high school students in India. Billions of people have spent time memorizing this formula for solving quadratic equations. However, according to Po-Shen Loh, a mathematician from Carnegie Mellon University, there is a faster, more effective way available, but it has remained hidden for a long time.
In his research paper, Loh said that the quadratic formula was a triumph of ancient mathematicians but it has some shortcomings.
Solving the quadratic equations with the old formula is a strenuous task that generations of students have had to do. However, that much effort might not be necessary. Some other alternatives are completing the square or factoring.
However, before this finding, the quadratic formula is considered the most reliable and comprehensive method.
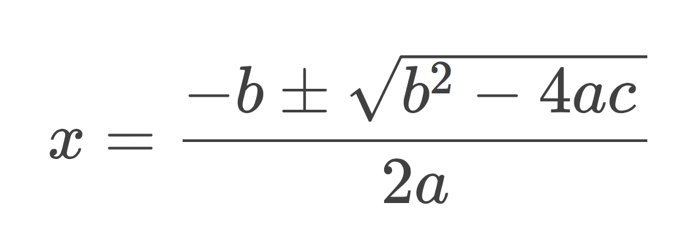
Back in September, when Loh was thinking about the mathematics that makes the quadratic equations, a simplified way to achieve the same result came to him. The best part is, the new formula is easy to remember.
Loh said that he was taken aback by the simplicity of the method and the fact that no textbook has ever mentioned it.
The old formula focuses on the two numbers making up c in ax2 + bx + c = 0 while Loh’s method leverages an averaging technique that emphasizes those that make up b. The former often requires some guesswork to find the correct answers.
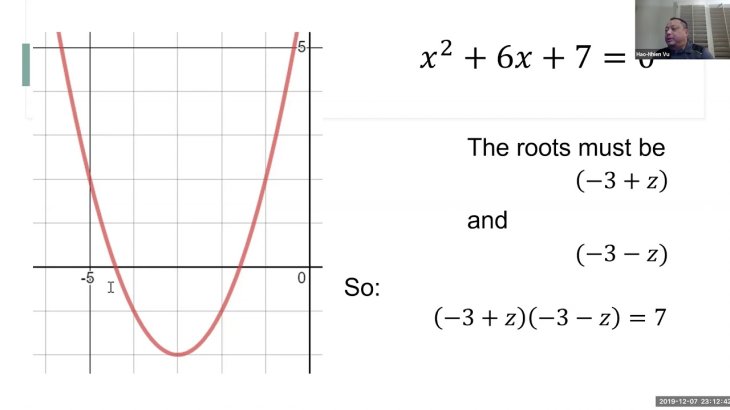
As stated by Loh on his website, if the sum is two, 1 is the average. Whatever the numbers, they are always 1 minus and plus an amount. What we have to do is to find z (making 1 + z and 1 – z) to make the two numbers. The z can be 0.
When you determine the value of z according to the alternative method of Loh, you can solve almost any quadratic equation’s roots, as long as some other conditions are met.
The author of the finding said that the method took into account steps found out by French, Babylonian, and Greek mathematicians. He is confident that it was unknown and never been taught before, as he found no evidence of its existence in English sources.
But after that, he found out about an article published in 1989 which described something quite similar to his finding. This proves that the new method is not actually something totally “new.”
Since this method is so simple and universal, it raises another question of why we have never heard of it before. With it, no one needs to memorize the quadratic formula ever again, instead, all we have to do is to remember a much simpler method.
So far, no one knows for sure this is the case but we are talking about a mass alteration of math textbooks, which can take some of the burdens off students’ shoulders. And we do not take textbooks changes lightly as it can affect millions of young minds all over the world.
For so long, many people have thought that mathematics is too hard and complicated for them, and Loh’s finding can be the first in a series of discoveries that can simplify the work. It might even ignite interest in math in many people.
The research paper can be found on arXiv.org. A more thorough explanation of how to solve quadratic equations in the new way can be found here.
>>> Top 10 Famous Indian Mathematicians In The World
Featured Stories

Features - Apr 09, 2025
EliteHubs Opens Computer Showroom in Pune with Extended Support Services

Review - Apr 05, 2025
Nintendo Switch 2 vs PlayStation 4, Which Is More Powerful?

Review - Apr 03, 2025
Top 5 Budget Cameras For Beginners (2025)

Features - Feb 26, 2025
Elon Musk Eyes Indian Market: Tesla’s Next Big Move?

Features - Aug 03, 2023
The Impact of Social Media on Online Sports Betting

Features - Jul 10, 2023
5 Most Richest Esports Players of All Time

Features - Jun 07, 2023
Is it safe to use a debit card for online gambling?

Features - May 20, 2023
Everything You Need to Know About the Wisconsin Car Bill of Sale

Features - Apr 27, 2023
How to Take Advantage of Guarantee Cashback in Online Bets

Features - Mar 08, 2023
0 Comments
Sort by Newest | Popular