Mathematical Secrets Behind 3,700-Year-Old Ancient Tablet Unlocked
Dhir Acharya - Jun 23, 2019

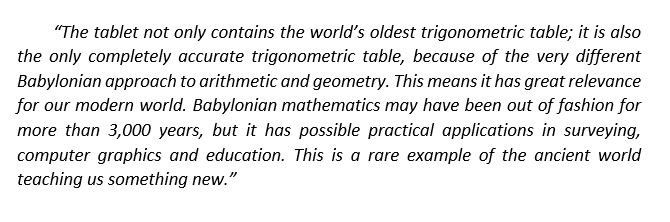
Called Primpton 322, the tablet represents the oldest and most accurate trigonometric table in the world that’s still working.
- Indian Kid Broke 4 World Records In Maths After Surviving Severe Head Injury
- A Person Who Is Good At Maths Might Have A Successful Career
- Fear Maths, Love Maths, You Will Find Yourself In These 12 Epic Bollywood Quotes
About a thousand years or more before the Pythagoras theorem, an unknown genius in Babylon had already used a clay tablet, a reed pen, with which he not only marked out the same theorem, but he also wrote a series of trigonometry tables that were claimed by scientists to be more accurate compared with today’s tables.
Scientists believe that they have cracked secrets behind the broken tablet, which is now 3,700 years old and stands in Columbia University’s collections.

According to the team from Sydney-based University of New South Wales, the tablet’s 15 rows and 4 columns of cuneiform, which are wedge-shaped indentations created in the wet clay, represent the oldest and most accurate trigonometric table in the world that’s still working. A trigonometric table is a working tool used for surveys and calculation in the construction of pyramids, palaces, and temples.
The Babylonian architecture and engineering are famous for their sophistication, born out by excavation. For example, some archaeologists believed that the Hanging Gardens of Babylon was a planted step pyramid featuring a sophisticated artificial watering system. Greek historians recognized it as one of the ancient world’s seven wonders.

Daniel Mansfield, school of mathematics and statistics, the University of New South Wales, described the tablet. This is likely to unlock some Babylonian methods as a fascinating work of math that illustrates undoubted genius, which may better help in the modern world as the Babylonian calculations’ use of base 60 allowed a lot more accurate fractions than the base 10 in Maths.
For almost a hundred years, there have been arguments among mathematicians about the interpretation of the Plimpton 322 tablet, which was bequeathed to Columbia University by the New York publisher George Plimpton in the 1930s. He bought the tablet from a flamboyant amateur archaeologist.
Mansfield and colleague Norman Wildberger published the research in the journal Historica Mathematica. Accordingly, for decades, mathematicians understood that the tablet indicates a theorem found before the Pythagoras Theorem; however, they had never agreed on what the tablet was meant to do.

According to the research, the mystery here is to find out the reason the ancient scribes conducted such a complex task to generate and sort the numbers carved on the tablet. As revealed by the research, in Plimpton 322, the shapes of right-angled triangles are described through unique ratio-based trigonometry instead of circles and angles. This fascinating mathematical work indicates undoubted genius.
In addition, the tablet long predates Hipparchus, the Greek astronomer, who was traditionally considered the father of trigonometry.
Wildberger said that Plimpton 322 appeared over 1,000 years before Hipparchus. The tablet brings about new possibilities for not only mathematics research but also mathematics education. With this tablet, we can also see more accurate and simpler trigonometry with absolute advantages over the contemporary one.
The pair believe that Babylonian maths has more for us to learn, which is still hidden in unstudied or untranslated tablets.

It is suggested by Mansfield and Wildberger that Plimpton 322’s mathematics show that it initially had 38 rows and 6 columns. They believe the tablet is not simply a teaching aid to check calculation, but it’s also a working tool. Mansfield stated that the tablet could be useful in conducting surveys or calculating in architecture to build step pyramids, temples, or palaces.
In 1945, Otto Neugebauer, an Austrian mathematician, and Abraham Sachs, his associate, became the first people to note that Plimpton 322 includes 15 pairs of numbers that form parts of Pygathorean triples: a, b, and c, the whole numbers which a squared plus b squared plus c squared. A famous example of a Pythagorean triple is 3, 4 and 5; however, the tablet often has significantly larger values. For instance, its first row indicates the triple 119, 120, and 169.
Featured Stories

Features - Apr 09, 2025
EliteHubs Opens Computer Showroom in Pune with Extended Support Services

Review - Apr 05, 2025
Nintendo Switch 2 vs PlayStation 4, Which Is More Powerful?

Review - Apr 03, 2025
Top 5 Budget Cameras For Beginners (2025)

Features - Feb 26, 2025
Elon Musk Eyes Indian Market: Tesla’s Next Big Move?

Features - Aug 03, 2023
The Impact of Social Media on Online Sports Betting

Features - Jul 10, 2023
5 Most Richest Esports Players of All Time

Features - Jun 07, 2023
Is it safe to use a debit card for online gambling?

Features - May 20, 2023
Everything You Need to Know About the Wisconsin Car Bill of Sale

Features - Apr 27, 2023
How to Take Advantage of Guarantee Cashback in Online Bets

Features - Mar 08, 2023
0 Comments
Sort by Newest | Popular